
Methods were explored to reduce the calculation time using similar hardware, and estimates were made of how to reduce the calculation time using a more sophisticated computer system. The calculation time for a single three-dimensional beam was approximately 20 min using a VAX/750 without an array processor. The agreement was generally found to be better than 1% on the central axis.
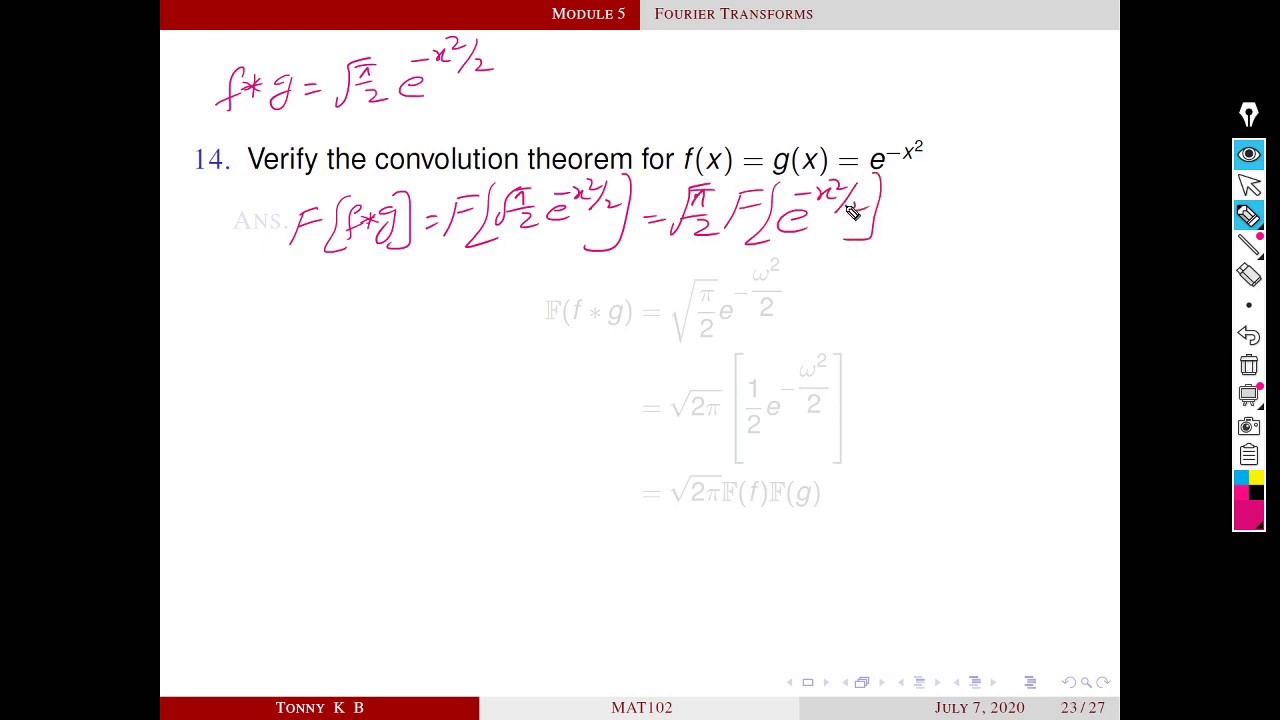
The dose distributions were compared with isodose distributions measured in a water phantom. The convolution integral is, in fact, directly related to the Fourier transform, and relies on a mathematical property of it. Dose distributions were obtained using FFT convolutions of the kernels for each energy with the spectrally weighted fluence distributions for that energy. The more subtle field-size effects of collimator scattering on the spectrum were obtained by calculating corrections to the spectral components using a least-squares search technique. The chapter also describes that Fourier series can be interpreted as expansion of a periodic function into sinusoidal functions. To consider applications of Fourier transform, we introduce a function corresponding to an impulse. The continuous bremsstrahlung spectrum was modeled using five discrete energies. The Fourier transform of a convolution integral gives us the product of Fourier transforms of each function. The EGS4 code was also used to estimate initially the spectrum by simulating the production, filtering, and flattening of the beam in the collimator of the linear accelerator. Convolution kernels were employed that had been calculated by Mackie using the EGS4 Monte Carlo code. Convolution concepts were implemented using the discrete fast Fourier transform (FFT) to model the three-dimensional dose distribution due to x-rays produced by a medical linear accelerator. As the Convolution Theorem18 states, convolution between two functions in the spatial domain corresponds to point-wise multiplication of the two functions in.


 0 kommentar(er)
0 kommentar(er)
